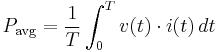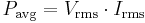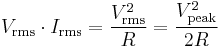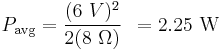Audio power
| Sound measurements |
|---|
| Sound pressure p, SPL |
| Particle velocity v, SVL |
| Particle displacement ξ |
| Sound intensity I, SIL |
| Sound power Pac |
| Sound power level SWL |
| Sound energy |
| Sound energy density E |
| Sound energy flux q |
| Acoustic impedance Z |
| Speed of sound c |
| Audio frequency AF |
Audio power is the electrical power transferred from an audio amplifier to a loudspeaker, measured in watts. The electrical power delivered to the loudspeaker, together with its sensitivity, determines the sound power level generated (with the rest being converted to heat).
Amplifiers are limited in the electrical energy they can amplify, while loudspeakers are limited in the electrical energy they can convert to sound energy without distorting the audio signal or destroying themselves. These power ratings are important to consumers finding compatible products and comparing competitors.
Power handling
In audio electronics, there are several methods of measuring power output (for such things as amplifiers) and power handling capacity (for such things as loudspeakers). The question has engineering, regulatory (consumer protection and advertising), and psychoacoustical aspects and is, in a serious sense, much more complex than may be imagined.
Amplifiers are valued in part by their power output capacity. And in the interest of being able to advertise a higher power output number, manufacturers in the US (and elsewhere) began to take advantage of the highly variable nature of most audio signals (especially musical sources) and to cite the peak output (quite brief and rarely sustainable for long) as the amplifier power. There being no standards, imaginative approaches came to be so common that the US Federal Trade Commission intervened in the market and required all amplifier manufacturers to use an engineering based measure (root-mean square) in addition to any other value they might cite.
Amplifiers, being electronic devices, have power limitations deriving from both their electrical and mechanical properties. All amplifiers produce heat as a byproduct of their operation, and if that heat is generated too fast, temperatures will rise high enough to damage components. In addition, for any given electrical load, higher power means higher voltage and current delivered, and either may exceed the capacity of one or more amplifier components.
There are no similar loudspeaker power handling measurement methods in the US; the problem is much harder as many loudspeaker systems have very different power handling capacities at different frequencies (eg, tweeters which handle high frequency signals are physically small and easily damaged, while woofers which handle low frequency signals are larger and more robust) in addition to the previously cited great variation in the power levels inherent in musical signals presented to a loudspeaker.
For loudspeakers, there is also a thermal and a mechanical aspect to maximum power handling. Not all energy delivered to a loudspeaker is emitted as sound. In fact, most is converted to heat, and that heat must not rise too high or damage will follow. High level signals over a prolonged period can cause thermal damage, some of which will be immediately obvious, but much will have the effect of reducing longevity or performance margin. In addition, loudspeaker components have mechanical limits which can be exceeded by even a very brief power peak; an example is the most common sort of loudspeaker driver, which cannot move in or out more than some limit without mechanical damage.
Power calculations
Since the instantaneous power of an AC waveform varies over time, AC power, which includes audio power, is typically measured as an average over time. It is based on this formula:[1]
For a purely resistive load, a simpler equation can be used, based on the root mean square (RMS) values of the voltage and current waveforms:
In the case of a steady sinusoidal tone (not music) into a purely resistive load, this can be calculated from the peak amplitude of the voltage waveform (which is easier to measure with an oscilloscope) and the load's resistance:
Though a speaker is not purely resistive, these equations are often used to approximate power measurements for such a system.
Example
An ideal (100% efficient) push-pull amplifier with a 12-volt supply can drive a sinusoidal signal with a peak amplitude of 6 V. When connected to an 8 ohm loudspeaker this would deliver:
Thus the output of an inexpensive car audio amplifier is limited by the voltage of the alternator. In most actual car systems, the amplifiers are connected in a bridge-tied load configuration, and speaker impedances are no higher than 4 Ω. High-power car amplifiers use a DC-to-DC converter to generate a higher supply voltage.
Continuous power
Continuous power ratings are a staple of performance specifications for audio amplifiers and, sometimes, loudspeakers. Continuous power is sometimes incorrectly referred to as RMS power and is derived from Root mean square (RMS), a method for measuring AC voltage or current.
In its 1974 Amplifier Rule meant to combat the unrealistic power claims made by many hi-fi amplifier manufacturers, the FTC prescribed continuous power measurements performed with sine wave signals on advertising and specification citations for amplifiers sold in the US. Typically, an amplifier's power specifications are calculated by measuring its RMS output voltage, with a continuous sine wave signal, at the onset of clipping—defined arbitrarily as a stated percentage of total harmonic distortion (THD)—into specified load resistances. Typical loads used are 8 and 4 ohms per channel; many amplifiers used in professional audio are also specified at 2 ohms.
Continuous power measurements do not actually describe the highly varied signals found in audio equipment but are widely regarded as a reasonable way of describing an amplifier's maximum output capability. Most amplifiers are capable of higher power if driven further into clipping, with corresponding increases in harmonic distortion, so the continuous power output rating cited for an amplifier should be understood to be the maximum power (at or below a particular acceptable amount of harmonic distortion) in the frequency band of interest. For audio equipment, this is nearly always the nominal frequency range of human hearing, 20Hz to 20 kHz.
In loudspeakers, thermal capacities of the voice coils and magnet structures largely determine continuous power handling ratings. However, at the lower end of a loudspeaker's usable frequency range, its power handling might necessarily be derated because of mechanical excursion limits. For example, a subwoofer rated at 100 watts may be able to handle 100 watts of power at 80 hertz, but at 25 hertz it might not be able to handle nearly as much power since such frequencies would, for some drivers in some enclosures, force the driver beyond its mechanical limits much before reaching 100 watts from the amplifier. The continuous ("RMS") value is also referred to as the nominal value, there being a regulatory requirement to use it.
Peak power
Peak power is the maximum level of work or energy output that is measured during an observation period. Peak power here refers to the maximum amount of power an electronic component can possibly handle for an instant without damage. Because of the highly dynamic nature of many audio signals (eg, music, which accounts for an alternative name, music power) there is some sense in attempting to characterize the ability of equipment to handle quickly changing power levels. But, how small an instant is a matter of some variation from observer to observer and so a peak power rating is necessarily more than a little indeterminate.
It always produces a higher value than the continuous ("RMS") figure, however, and so has been tempting to use in advertising. Generally, whatever the definition of instant used, distortion is also higher for an instant. For instance, an amplifier (especially a surround sound receiver), may be rated at 1,000 watts peak power, but the harmonic distortion level might be 10 percent under those conditions. Peak power is also referred to as max power or PMPO (Peak Music Power Output).[2] It is often five or six times greater than the continuous ("RMS") rating.
Ambiguity: Among amplifiers, the peak power rating is fairly ambiguous as it varies depending on "acceptable" maximum harmonic distortion. For example, the peak power output rating of surround sound receivers is often taken at 10 percent THD. The highest generally acceptable level of total harmonic distortion is considered to be 0.1%. Hence, two max power output ratings are sometimes provided, one at 0.1% THD, and another at 10% THD.
Total system power
Total system power is a term often used in audio electronics to rate the power of an audio system. Total system power refers to the total power consumption of the unit, rather than the power handling of the speakers or the power output of the amplifier. This can be viewed as a somewhat deceptive marketing ploy, as the total power consumption of the unit will of course be greater than any of its other power ratings, except for, perhaps, the peak power of the amplifier, which is essentially an exaggerated value anyway. Shelf stereos and surround sound receivers are often rated using total system power.
One way to use total system power to get a more accurate estimate of power is to consider the amplifier class which would give an educated guess of the power output by considering the efficiency of the class. For example, class AB amplifiers are around 25 or 50% efficiency while Class D amps are much higher; around 80% or more efficiency. A very exceptional efficiency for a specific Class D amp, the ROHM BD5421efs, operates at 90% efficiency.[3]
In some cases, an audio device may be measured by the total system power of all its loudspeakers by adding all their peak power ratings. Many home theater in a box systems are rated this way. Often low-end home theater systems' power ratings are taken at a high level of harmonic distortion as well; as high as 10%, which would be noticeable.[4]
Sine wave power
The term sine power is used in the specification and measurement of audio power. A meaningful and reliable measure of the maximum power output of an audio amplifier – or the power handling of a loudspeaker – is continuous average sine wave power. The peak power of a sine wave of RMS value X is √2*X; conversely, the RMS value of a sine wave of peak X is (1/√2)*X. For a resistive load, the average power is the product of the RMS current and RMS voltage.
Harmonic distortion increases with power output; the maximum continuous power output of an amplifier is always stated at a given percentage of distortion, say 1% THD+N at 1 kHz. Considerably more power can be delivered if distortion is allowed to increase; some manufacturers quote maximum power at a higher distortion, like 10%, making their equipment appear more powerful than if measured at an acceptable distortion level.
In the US on May 3, 1974, the Amplifier Rule CFR 16 Part 432 (39 FR 15387) was instated by the Federal Trade Commission (FTC) requiring audio power and distortion ratings for home entertainment equipment to be measured in a defined manner with power stated in RMS terms. (See more in the section Standards at the end of this article). The erroneous term "watts RMS" is actually used in CE regulations.[5]
PMPO
Peak Music Power Output (PMPO), sometimes misused in advertising as Peak momentary performance output, is a much more dubious figure of merit, of interest more to advertising copy-writers than to consumers. The term PMPO has never been defined in any standard, but it is often taken to be the sum of some sort of peak power for each amplifier in a system. Different manufacturers use different definitions, so that the ratio of PMPO to continuous power output varies widely; it is not possible to convert from one to the other. Most amplifiers can sustain their PMPO for only a very short time, if at all; loudspeakers are not designed to withstand their stated PMPO for anything but a momentary peak without serious damage.
Power and loudness in the real world
Perceived "loudness" varies logarithmically with output power (other inversely proportionate factors are; frequency, number and material of objects through which the sound waves must travel, as well as distance between source and receiver) a given change in output power produces a much smaller change in perceived loudness. Consequently it is useful and accurate to express perceived loudness in the logarithmic decibel (dB) scale.
An increase/decrease of 3 dB corresponds to a doubling/halving of power. The sensitivity of loudspeakers,rather than merely the often-quoted power-handling capacity, is important. Many high quality domestic speakers have a sensitivity of 84 dB for 1 W at 1 meter, but professional speakers can have a figure of 90 dB for 1 W or even 100 dB (especially for some large-coned woofers). I.E., An '84 dB' source "speaker" would require a 400-watt amplifier to produce the same audio energy as a '90 dB' source being driven by a 100-watt amplifier, or a '100 dB' source being driven by a 9.92 watt amplifier. This does not mean a bigger speaker can produce more sound with less overall power. Just that a larger speaker can typically handle more initial power and so requires less amplification to achieve the same high level of output. This means using a speaker with a higher dB rating can be more advantageous as very high power amplifiers become impractical.
A better measure of the 'power' of a system is therefore a plot of maximum loudness before clipping of the amplifier and loudspeaker combined, in dB SPL, at the listening position intended, over the audible frequency spectrum. A good system should be capable of generating higher sound levels below 100 Hz before clipping, as the human ear is less sensitive to low frequencies, as indicated by Equal-loudness contours.
'Music power' — the real issues
The term "Music Power" has been used in relation to both amplifiers and loudspeakers with some validity. When live music is recorded without amplitude compression or limiting, the resulting signal contains brief peaks of very much higher amplitude (20 dB or more) than the mean, and since power is proportional to the square of signal voltage their reproduction would require an amplifier capable of providing brief peaks of power around a hundred times greater than the average level. Thus the ideal 100-watt audio system would need to be capable of handling brief peaks of 10,000 watts in order to avoid clipping (see Programme levels). Most loudspeakers are in fact capable of withstanding peaks of several times their continuous rating (though not a hundred times), since thermal inertia prevents the voice coils from burning out on short bursts. It is therefore acceptable, and desirable, to drive a loudspeaker from a power amplifier with a higher continuous rating several times the steady power that the speaker can withstand, but only if care is taken not to overheat it; this is difficult, especially on modern recordings which tend to be heavily compressed and so can be played at high levels without the obvious distortion that would result from an uncompressed recording when the amplifier started clipping.
An amplifier can be designed with an audio output circuitry capable of generating a certain power level, but with a power supply unable to supply sufficient power for more than a very short time, and with heat sinking that will overheat dangerously if full output power is maintained for long. This makes good technical and commercial sense, as the amplifier can handle music with a relatively low mean power, but with brief peaks; a high 'music power' output can be advertised (and delivered), and money saved on the power supply and heat sink. Program sources that are significantly compressed are more likely to cause trouble, as the mean power can be much higher for the same peak power. Circuitry which protects the amplifier and power supply can prevent equipment damage in the case of sustained high power operation.
More sophisticated equipment usually used in a professional context has advanced circuitry which can handle high peak power levels without delivering more average power to the speakers than they and the amplifier can handle safely.
Matching amplifier to loudspeaker
Charles "Chuck" McGregor, while serving as senior technologist for Eastern Acoustic Works, wrote a guideline for professional audio purchasers wishing to select properly-sized amplifiers for their loudspeakers. Chuck McGregor recommended a rule of thumb in which the amplifier's maximum power output rating was twice the loudspeaker's continuous (so-called "RMS") rating, give or take 20%. In his example, a loudspeaker with a continuous power rating of 250 watts would be well-matched by an amplifier with a maximum power output within the range of 400 to 625 watts.[6]
Power handling in 'active' speakers
Active speakers comprise two or three speakers per channel, each fitted with its own amplifier, and preceded by an electronic crossover filter to separate the low-level audio signal into the frequency bands to be handled by each speaker. This approach enables complex active filters to be used on the low level signal, without the need to use passive crossovers of high power handling capability but limited rolloff and with large and expensive inductors and capacitors. An additional advantage is that peak power handling is greater if the signal has simultaneous peaks in two different frequency bands. A single amplifier has to handle the peak power when both signal voltages are at their crest; as power is proportional to the square of voltage, the peak power when both signals are at the same peak voltage is proportional to the square of the sum of the voltages. If separate amplifiers are used, each must handle the square of the peak voltage in its own band. For example, if bass and midrange each has a signal corresponding to 10 W of output, a single amplifier capable of handling a 40 W peak would be needed, but a bass and a treble amplifier each capable of handling 10 W would be sufficient. This is relevant when peaks of comparable amplitude occur in different frequency bands, as with wideband percussion and high-amplitude bass notes.
For most audio applications more power is needed at low frequencies. This requires a high-power amplifier for low frequencies (e.g., 200 watts for 20–200 Hz band), lower power amplifier for the midrange (e.g., 50 watts for 200 to 1000 Hz), and even less the high end (e.g. 5 watts for 1000–20000 Hz). Proper design of a bi/tri amplifier system requires a study of driver (speaker) frequency response and sensitivities to determine optimal crossover frequencies and power amplifier powers.
Regional Variations
US
Peak momentary power output and peak music power output are two different measurements with different specifications and should not be used interchangeably. Manufacturers who use different words such as pulse or performance may be reflecting their own non-standard system of measurement, with an unknown meaning. The Federal Trade Commission is putting an end to this with Federal Trade Commission (FTC) Rule 46 CFR 432 (1974), affecting Power Output Claims for Amplifiers Utilized in Home Entertainment Products.
In response to a Federal Trade Commission order, the Consumer Electronics Association has established a clear and concise measure of audio power for consumer electronics. They have posted an FTC approved product marking template on their web site and the full standard is available for a fee. Many believe this will resolve much of the ambiguity and confusion in amplifier ratings. There will be ratings for speaker and powered speaker system too. This specification only applies to audio amplifiers. A UE counterpart is expected and all equipment sold in the US and Europe will be identically tested and rated.[7]
On May 3, 1974, the Amplifier Rule CFR 16 Part 432[8] was instated by the Federal Trade Commission (FTC) requiring audio power and distortion ratings for home entertainment equipment to be measured in a defined manner with power stated in RMS terms. This rule was amended in 1998 to cover self-powered speakers such as are commonly used with personal computers (see examples below).
This regulation did not cover automobile entertainment systems, which consequently still suffer from power ratings confusion. However, a new Approved American National Standard ANSI/CEA-2006-B which includes testing & measurement methods for mobile audio amplifiers is being slowly phased into the market by many manufacturers.[9]
Europe
DIN (Deutsches Institut für Normung, German Institute for Standardization) describes in DIN 45xxx several standards for measuring audio power. The DIN-standards (DIN-norms) are in common use in Europe.[10]
International
IEC 60268-2 defines power amplifier specifications including power output.[11]
See also
References
- ^ Average Power in an AC Circuit – Richard Vawter
- ^ http://www.audioholics.com/education/amplifier-technology/amplifier-power-ratings
- ^ http://www.eetasia.com/ART_8800464222_499501_NP_c0bbb082.HTM
- ^ http://hometheater.about.com/cs/audiocomponents/a/aapowermada.htm
- ^ CEA-2006-A, Mobile Amplifier Power, http://www.ce.org/Standards/3108.asp, retrieved 2011-08-13
- ^ ProSoundWeb, Study Hall. Chuck McGregor, How Many Watts : Amps vs. Loudspeakers: The eternal question answered - what's the "right" wattage for my loudspeakers. Retrieved February 27, 2009.
- ^ CEA-490-A: Test Methods of Measurement for Audio Amplifiers, Federal Trade Commission (FTC) Rule, Power Output Claims for Amplifiers Utilized in Home Entertainment Products, 46 CFR 432 (1974). Accessed 2011-08-13.
- ^ 39 FR 15387, http://cfr.law.cornell.edu/cfr/cfr.php?title=16&type=part&value=432
- ^ "CEA Standard for testing mobile audio equipment". http://www.ce.org/Standards/browseByCommittee_2648.asp. Retrieved 2011-08-13.
- ^ "Understanding amplifier power ratings". http://www.epanorama.net/documents/audio/amplifier_power.html. Retrieved 2011-08-13.
- ^ "IEC 60268-2 (preview)". IEC. 2008-08. http://webstore.iec.ch/preview/info_iec60268-3%7Bed3.0%7Den.pdf. Retrieved 2011-08-24.
External links
- Amplifier Power Ratings (and How to calculate satisfactory PMPO values) by Rod Elliott
- Understanding amplifier power ratings
- Audio power and the corresponding factors: Subjectivly sensed loudness (volume), objectively measured sound pressure (voltage), and theoretically calculated sound intensity (acoustic power)